|
|
 |
 |
 |
 |
 |
|
|
 |
 |
 |
 |
 |
Course webpages
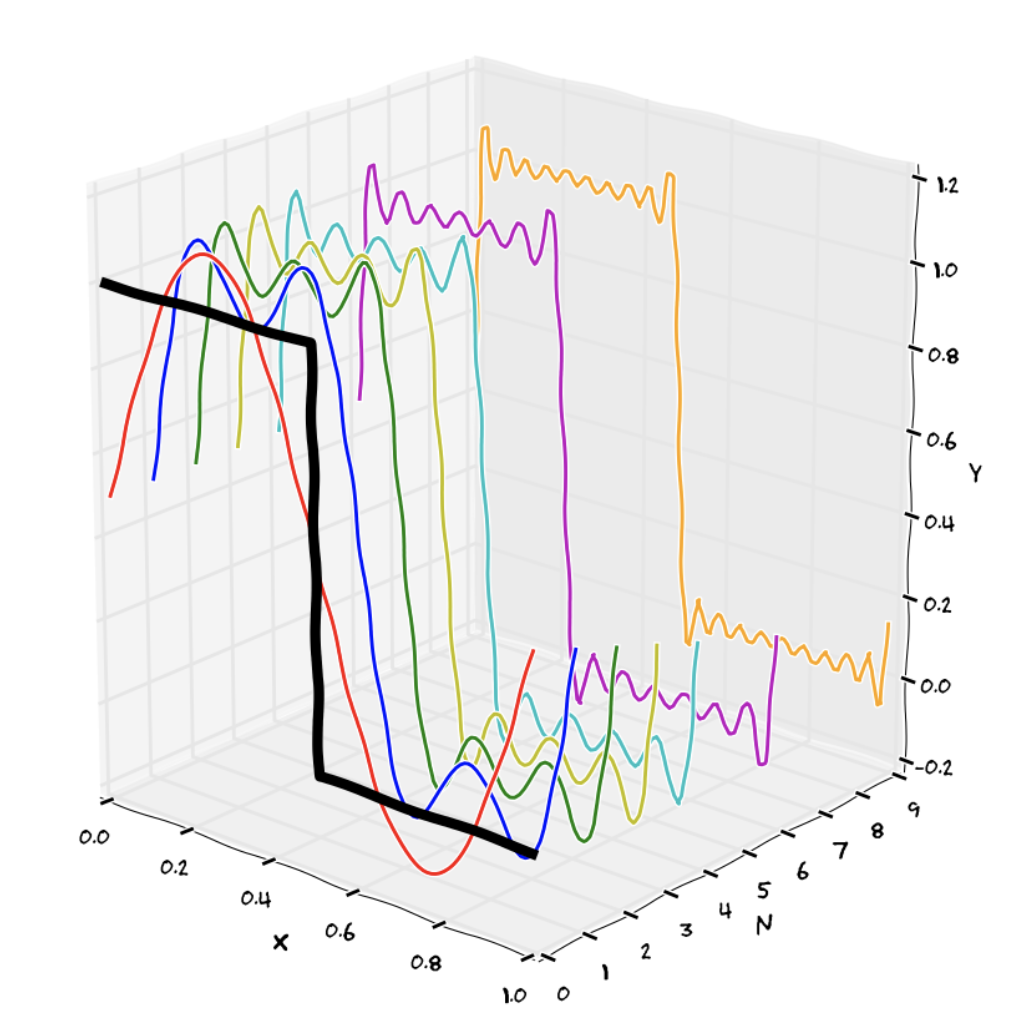
Fourier Analysis
Instructors
Dr Das, Dr Salak
Availability
2nd year in Department of Physics
Overview
The introductory course on Fourier analysis covers the basics of Fourier series (Dirichlet conditions, Fourier coefficients, Parseval’s theorem), Fourier transform and its properties, convolution, Dirac delta function, and Laplace transform.
Complex analysis: Complex numbers, Complex differentiation, Cauchy’s Integral Theorem, Laurent Expansion, Residue Theorem, Calculus of Residues, Cauchy Principal Value, Mittag-Leffler’s Expansion Theorem, Integral Applications.